Hi, this is Min and I will introduce the Fuel-Cost function today. This function is very useful for calculating the economic results of power system planning. I hope this will help you improve your further research on this matter.
The fuel-cost function is a mathematical expression that represents the cost of generating electricity as a function of the amount of electricity generated. It is an essential tool for power plant operators and electricity market participants, as it helps them to optimize their operations and make informed decisions about how to purchase and sell electricity.
The fuel-cost function is typically quadratic, meaning that it is represented by a second-degree polynomial equation. This is because the cost of generating electricity increases at an increasing rate as more electricity is generated. This is due to several factors, including the fact that power plants must burn more fuel to generate more electricity and that the efficiency of power plants decreases as they are operated at higher output levels.
The specific form of the fuel-cost function will vary depending on the type of power plant and the type of fuel being used. However, the general form of the function is as follows:
C(P) = aP^2 + bP + c
where:
- C(P) is the fuel cost in dollars
- P is the amount of electricity generated in megawatt-hours (MWh)
- a, b, and c are coefficients that are specific to the power plant and the type of fuel being used
The coefficient a is the most important in the fuel-cost function, as it represents the incremental cost of generating electricity. This is the cost of generating one additional megawatt-hour of electricity, assuming that all other factors, such as fuel prices and power plant efficiency, remain constant.
The coefficients b and c are also important, as they represent the fixed costs of generating electricity. These costs are independent of the amount of electricity generated, and they include things like the cost of maintaining the power plant and the cost of financing its construction.
The fuel-cost function can be used to calculate the cost of generating electricity at any given output level. It can also be used to optimize power plant operations by minimizing the total fuel cost. Additionally, the fuel-cost function is used in electricity market models to simulate the behavior of market participants and to determine the price of electricity.
Here is an example of how to use the fuel-cost function to calculate the cost of generating electricity at a given output level. Suppose that the fuel-cost function for a power plant is as follows:
C(P) = 0.002P^2 + 5P + 100
To calculate the cost of generating 100 MWh of electricity, we would substitute P = 100 into the fuel-cost function:
C(100) = 0.002(100)^2 + 5(100) + 100 = 550
Therefore, the cost of generating 100 MWh of electricity at this power plant would be $550.
I am also giving another example below.
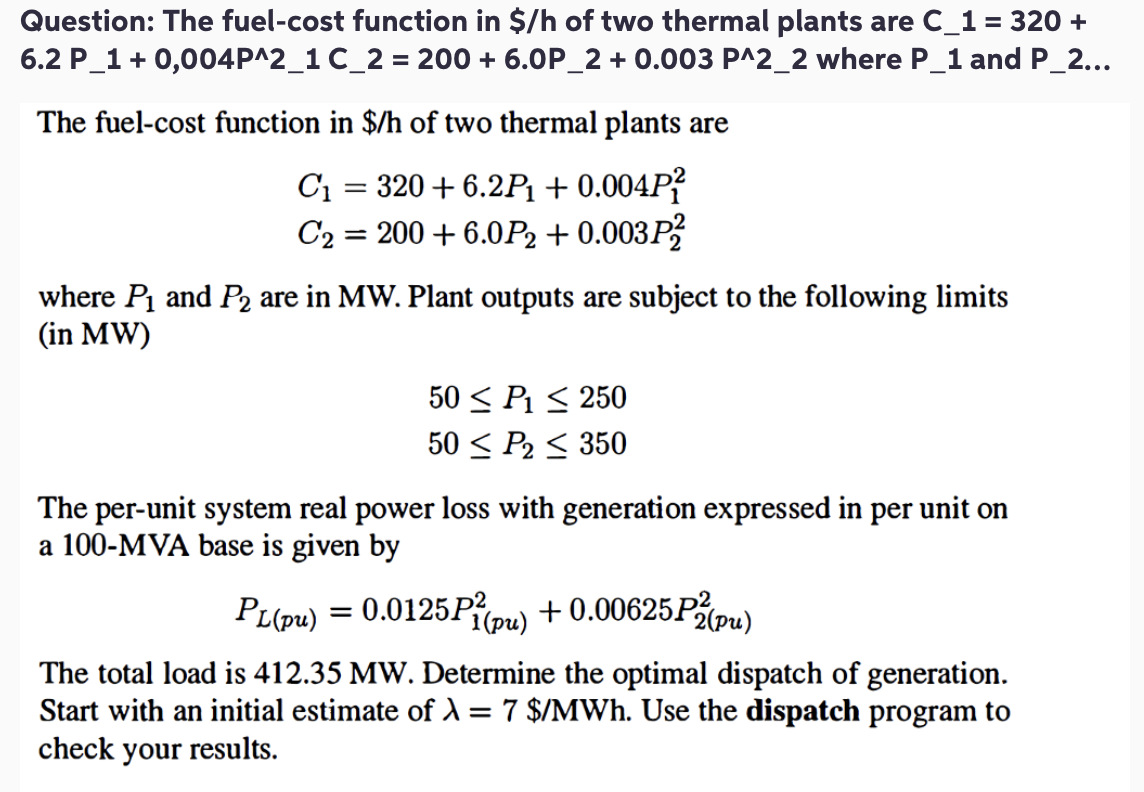
I hope this explanation is helpful. Please let me know if you have any other questions.
'전기 엔지니어링(Electrical Engineering)' 카테고리의 다른 글
| 주상변압기 스펙, 도면, 공급업체, 구조 그리고 결선도 (2) | 2023.12.13 |
|---|---|
| What is BESS?? Key components and Functions (2) | 2023.12.06 |
| kW vs kWh: What's the difference? (3) | 2023.11.21 |
| 전력도매시장과 소매전력시장의 차이점 (6) | 2023.11.19 |
| What is the Cycling cost in the power industry?? (7) | 2023.10.30 |